Determine 쑠w of These Two Cells After They Have Again Come to Equilibrium With Each Other.

Chapter 13. Fundamental Equilibrium Concepts

13.4 Equilibrium Calculations

Learning Objectives

By the end of this section, you will be able to:

• Write equations representing changes in concentration and pressure for chemic species in equilibrium systems
• Apply algebra to perform various types of equilibrium calculations

Nosotros know that at equilibrium, the value of the reaction quotient of any reaction is equal to its equilibrium constant. Thus, we tin use the mathematical expression for Q to make up one's mind a number of quantities associated with a reaction at equilibrium or budgeted equilibrium. While we have learned to identify in which direction a reaction will shift to reach equilibrium, we want to extend that agreement to quantitative calculations. We do then past evaluating the ways that the concentrations of products and reactants modify equally a reaction approaches equilibrium, keeping in mind the stoichiometric ratios of the reaction. This algebraic approach to equilibrium calculations will be explored in this section.

Changes in concentrations or pressures of reactants and products occur as a reaction system approaches equilibrium. In this section nosotros will come across that we can chronicle these changes to each other using the coefficients in the balanced chemical equation describing the system. We use the decomposition of ammonia as an case.

On heating, ammonia reversibly decomposes into nitrogen and hydrogen according to this equation:

[latex]2\text{NH}_3(thousand)\;{\rightleftharpoons}\;\text{N}_2(1000)\;+\;3\text{H}_2(g)[/latex]

If a sample of ammonia decomposes in a closed system and the concentration of N₂ increases by 0.11 M, the change in the North_two concentration, Δ[N_ii], the final concentration minus the initial concentration, is 0.11 M. The modify is positive considering the concentration of North₂ increases.

The change in the H₂ concentration, Δ[H₂], is likewise positive—the concentration of H₂ increases as ammonia decomposes. The chemic equation tells united states of america that the modify in the concentration of H_two is three times the change in the concentration of N₂ because for each mole of N₂ produced, 3 moles of H_ii are produced.

[latex]{\Delta}[\text{H}_2] = 3\;\times\;{\Delta}[\text{North}_2][/latex]

[latex]= iii\;\times\;(0.11\;Thou) = 0.33\;Chiliad[/latex]

The change in concentration of NH_three, Δ[NH₃], is twice that of Δ[Due north_two]; the equation indicates that 2 moles of NH₃ must decompose for each mole of N₂ formed. Withal, the change in the NH₃ concentration is negative because the concentration of ammonia decreases as it decomposes.

[latex]{\Delta}[\text{NH}_3] = -2\;\times\;{\Delta}[\text{N}_2] = -2\;\times\;(0.11\;M) = -0.22\;M[/latex]

We can relate these relationships directly to the coefficients in the equation

[latex]\brainstorm{array}{ccccc} two\text{NH}_3(g) & {\rightleftharpoons} & \text{Due north}_2(g) & + & 3\text{H}_2(g) \\[0.5em] {\Delta}[\text{NH}_3] = -2\;\times\;{\Delta}[\text{Northward}_2] & & {\Delta}[\text{N}_2] = 0.xi\;Thou & & {\Delta}[\text{H}_2] = 3\;\times\;{\Delta}[\text{N}_2] \end{array}[/latex]

Note that all the changes on one side of the arrows are of the aforementioned sign and that all the changes on the other side of the arrows are of the opposite sign.

If we did not know the magnitude of the change in the concentration of Due north₂, nosotros could represent it past the symbol x.

[latex]{\Delta}[\text{N}_2] = x[/latex]

The changes in the other concentrations would then be represented as:

[latex]{\Delta}[\text{H}_2] = 3\;\times\;{\Delta}[\text{North}_2] = 3x[/latex]

[latex]{\Delta}[\text{NH}_3] = -2\;\times\;{\Delta}[\text{N}_2] = -2x[/latex]

The coefficients in the Δ terms are identical to those in the balanced equation for the reaction.

[latex]\begin{array}{ccccc} 2\text{NH}_3(g) & {\rightleftharpoons} & \text{N}_2(g) & + & three\text{H}_2(k) \\[0.5em] -2x & & x & & 3x \end{array}[/latex]

The simplest style for u.s.a. to find the coefficients for the concentration changes in any reaction is to use the coefficients in the balanced chemic equation. The sign of the coefficient is positive when the concentration increases; it is negative when the concentration decreases.

Case 1

Determining Relative Changes in Concentration
Consummate the changes in concentrations for each of the following reactions.

(a) [latex]\begin{assortment}{lcccc} \text{C}_2\text{H}_2(g) & + & 2\text{Br}_2(g) & {\rightleftharpoons} & \text{C}_2\text{H}_2\text{Br}_4(grand) \\[0.5em] x & & \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} \finish{array}[/latex]

(b) [latex]\brainstorm{array}{lcccc} \text{I}_2(aq) & + & \text{I}^{-}(aq) & {\rightleftharpoons} & \text{I}_3^{\;\;-}(aq) \\[0.5em] \rule[0ex]{two.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & x \end{array}[/latex]

(c) [latex]\begin{assortment}{lcccccc} \text{C}_3\text{H}_8(grand) & + & 5\text{O}_2(g) & {\rightleftharpoons} & 3\text{CO}_2(yard) & + & four\text{H}_2\text{O}(1000) \\[0.5em] x & & \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{ii.5em}{0.1ex} & & \rule[0ex]{two.5em}{0.1ex} \end{array}[/latex]

Solution
(a) [latex]\begin{assortment}{lcccc} \text{C}_2\text{H}_2(thou) & + & 2\text{Br}_2(k) & {\rightleftharpoons} & \text{C}_2\text{H}_2\text{Br}_4(yard) \\[0.5em] x & & 2x & & -10 \end{array}[/latex]

(b) [latex]\begin{array}{lcccc} \text{I}_2(aq) & + & \text{I}^{-}(aq) & {\rightleftharpoons} & \text{I}_3^{\;\;-}(aq) \\[0.5em] -ten & & -x & & x \end{array}[/latex]

(c) [latex]\begin{array}{lcccccc} \text{C}_3\text{H}_8(thou) & + & 5\text{O}_2(m) & {\rightleftharpoons} & three\text{CO}_2(g) & + & 4\text{H}_2\text{O}(g) \\[0.5em] 10 & & 5x & & -3x & & -4x \terminate{array}[/latex]

Bank check Your Learning
Complete the changes in concentrations for each of the post-obit reactions:

(a) [latex]\begin{array}{lcccc} ii\text{And so}_2(g) & + & \text{O}_2(grand) & {\rightleftharpoons} & 2\text{And so}_3(g) \\[0.5em] \rule[0ex]{2.5em}{0.1ex} & & 10 & & \dominion[0ex]{2.5em}{0.1ex} \end{array}[/latex]

(b) [latex]\begin{array}{lcc} \text{C}_4\text{H}_8(g) & {\rightleftharpoons} & two\text{C}_2\text{H}_4(1000) \\[0.5em] \rule[0ex]{two.5em}{0.1ex} & & -2x \end{array}[/latex]

(c) [latex]\begin{array}{lcccccc} 4\text{NH}_3(g) & + & 7\text{H}_2\text{O}(g) & {\rightleftharpoons} & 4\text{NO}_2(g) & + & 6\text{H}_2\text{O}(chiliad) \\[0.5em] \rule[0ex]{two.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{ii.5em}{0.1ex} \finish{array}[/latex]

Answer:

(a) iix, x, −2x; (b) x, −2x; (c) 4ten, 710, −ivx, −half dozenx or −ivx, −7x, 4x, 610

Calculations Involving Equilibrium Concentrations

Because the value of the reaction caliber of any reaction at equilibrium is equal to its equilibrium constant, we can utilize the mathematical expression for Q_c (i.due east., the law of mass action) to decide a number of quantities associated with a reaction at equilibrium. It may aid if nosotros keep in mind that Q_c = 1000_c (at equilibrium) in all of these situations and that there are only three basic types of calculations:

1. Adding of an equilibrium constant. If concentrations of reactants and products at equilibrium are known, the value of the equilibrium constant for the reaction can exist calculated.
2. Adding of missing equilibrium concentrations. If the value of the equilibrium constant and all of the equilibrium concentrations, except ane, are known, the remaining concentration can be calculated.
3. Adding of equilibrium concentrations from initial concentrations. If the value of the equilibrium constant and a fix of concentrations of reactants and products that are not at equilibrium are known, the concentrations at equilibrium can be calculated.

A similar list could be generated using Q_P , Thousand_P , and partial pressure. We will look at solving each of these cases in sequence.

Adding of an Equilibrium Constant

Since the law of mass action is the but equation we have to depict the relationship between Thousand_c and the concentrations of reactants and products, whatever problem that requires us to solve for 1000_c must provide enough data to determine the reactant and product concentrations at equilibrium. Armed with the concentrations, nosotros can solve the equation for K_c , as it volition exist the only unknown.

Example 2 showed united states how to determine the equilibrium constant of a reaction if we know the concentrations of reactants and products at equilibrium. The following instance shows how to employ the stoichiometry of the reaction and a combination of initial concentrations and equilibrium concentrations to determine an equilibrium constant. This technique, commonly chosen an ICE chart—for Initial, Change, and Equilibrium–will exist helpful in solving many equilibrium problems. A chart is generated get-go with the equilibrium reaction in question. Underneath the reaction the initial concentrations of the reactants and products are listed—these conditions are usually provided in the problem and nosotros consider no shift toward equilibrium to have happened. The next row of data is the modify that occurs equally the system shifts toward equilibrium—exercise not forget to consider the reaction stoichiometry as described in a previous section of this chapter. The concluding row contains the concentrations once equilibrium has been reached.

Example 2

Calculation of an Equilibrium Constant
Iodine molecules react reversibly with iodide ions to produce triiodide ions.

[latex]\text{I}_2(aq)\;+\;\text{I}^{-}(aq)\;{\rightleftharpoons}\;\text{I}_3^{\;\;-}(aq)[/latex]

If a solution with the concentrations of I₂ and I⁻ both equal to one.000 × 10⁻³ G before reaction gives an equilibrium concentration of I_two of vi.61 × 10⁻⁴ M, what is the equilibrium constant for the reaction?

Solution
We volition begin this problem by calculating the changes in concentration as the organization goes to equilibrium. And so we determine the equilibrium concentrations and, finally, the equilibrium abiding. Showtime, we ready a table with the initial concentrations, the changes in concentrations, and the equilibrium concentrations using −x every bit the modify in concentration of I_ii.

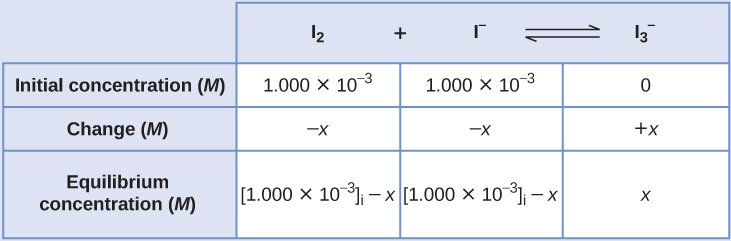
Since the equilibrium concentration of I₂ is given, we tin can solve for ten. At equilibrium the concentration of I_two is six.61 × 10⁻⁴ M so that

[latex]1.000\;\times\;10^{-iii}\;-\;10 = half-dozen.61\;\times\;x^{-4}[/latex]

[latex]x = one.000\;\times\;ten^{-3}\;-\;6.61\;\times\;10^{-4}[/latex]

[latex]= 3.39\;\times\;x^{-four}\;M[/latex]

Now nosotros tin fill in the table with the concentrations at equilibrium.

We now calculate the value of the equilibrium constant.

[latex]K_c = Q_c = \frac{[\text{I}_3^{\;\;-}]}{[\text{I}_2][\text{I}^{-}]}[/latex]

[latex]= \frac{3.39\;\times\;ten^{-4}\;One thousand}{(6.61\;\times\;10^{-4}\;Chiliad)(6.61\;\times\;x^{-4}\;M)} = 776[/latex]

Check Your Learning
Ethanol and acerb acid react and form water and ethyl acetate, the solvent responsible for the odor of some nail polish removers.

[latex]\text{C}_2\text{H}_5\text{OH}\;+\;\text{CH}_3\text{CO}_2\text{H}\;{\rightleftharpoons}\;\text{CH}_3\text{CO}_2\text{C}_2\text{H}_5\;+\;\text{H}_2\text{O}[/latex]

When 1 mol each of C₂H_vOH and CH₃CO_twoH are allowed to react in 1 50 of the solvent dioxane, equilibrium is established when 1313 mol of each of the reactants remains. Summate the equilibrium abiding for the reaction. (Note: Water is not a solvent in this reaction.)

Adding of a Missing Equilibrium Concentration

If nosotros know the equilibrium constant for a reaction and know the concentrations at equilibrium of all reactants and products except one, we can summate the missing concentration.

Example three

Calculation of a Missing Equilibrium Concentration
Nitrogen oxides are air pollutants produced past the reaction of nitrogen and oxygen at high temperatures. At 2000 °C, the value of the equilibrium constant for the reaction, [latex]\text{Northward}_2(grand)\;+\;\text{O}_2(g)\;{\rightleftharpoons}\;2\text{NO}(g)[/latex], is iv.1 × x⁻⁴. Find the concentration of NO(g) in an equilibrium mixture with air at 1 atm pressure at this temperature. In air, [N_two] = 0.036 mol/L and [O₂] 0.0089 mol/L.

Solution
We are given all of the equilibrium concentrations except that of NO. Thus, we can solve for the missing equilibrium concentration by rearranging the equation for the equilibrium constant.

[latex]K_c = Q_c = \frac{[\text{NO}]^2}{[\text{N}_2][\text{O}_2]}[/latex]

[latex][\text{NO}]^two = K_c[\text{North}_2][\text{O}_2][/latex]

[latex][\text{NO}] = \sqrt{K_c[\text{North}_2][\text{O}_2]}[/latex]

[latex]= \sqrt{(4.1\;\times\;10^{-4})(0.036)(0.0089)}[/latex]

[latex]= \sqrt{1.31\;\times\;ten^{-seven}}[/latex]

[latex]= 3.6\;\times\;10^{-4}[/latex]

Thus [NO] is iii.vi × 10⁻⁴ mol/L at equilibrium under these atmospheric condition.

Nosotros can cheque our answer by substituting all equilibrium concentrations into the expression for the reaction quotient to see whether it is equal to the equilibrium abiding.

[latex]Q_c = \frac{[\text{NO}]^2}{[\text{North}_2][\text{O}_2]}[/latex]

[latex]= \frac{(3.6\;\times\;10^{-4})^2}{(0.036)(0.0089)}[/latex]

[latex]Q_c = iv.0\;\times\;10^{-4} = K_c[/latex]

The answer checks; our calculated value gives the equilibrium constant within the error associated with the significant figures in the problem.

Check Your Learning
The equilibrium constant for the reaction of nitrogen and hydrogen to produce ammonia at a certain temperature is 6.00 × ten^−two. Calculate the equilibrium concentration of ammonia if the equilibrium concentrations of nitrogen and hydrogen are 4.26 One thousand and two.09 Grand, respectively.

Calculation of Changes in Concentration

If nosotros know the equilibrium constant for a reaction and a prepare of concentrations of reactants and products that are non at equilibrium, we can calculate the changes in concentrations every bit the system comes to equilibrium, as well equally the new concentrations at equilibrium. The typical process can be summarized in four steps.

1. Determine the direction the reaction proceeds to come to equilibrium.
   1. Write a balanced chemical equation for the reaction.
   2. If the direction in which the reaction must proceed to achieve equilibrium is not obvious, calculate Q_c from the initial concentrations and compare to K_c to make up one's mind the management of modify.
2. Determine the relative changes needed to attain equilibrium, then write the equilibrium concentrations in terms of these changes.
   1. Ascertain the changes in the initial concentrations that are needed for the reaction to reach equilibrium. Generally, nosotros represent the smallest change with the symbol x and express the other changes in terms of the smallest modify.
   2. Define missing equilibrium concentrations in terms of the initial concentrations and the changes in concentration determined in (a).
3. Solve for the change and the equilibrium concentrations.
   1. Substitute the equilibrium concentrations into the expression for the equilibrium constant, solve for x, and cheque whatsoever assumptions used to find x.
   2. Calculate the equilibrium concentrations.
4. Check the arithmetic.
   1. Check the calculated equilibrium concentrations by substituting them into the equilibrium expression and determining whether they give the equilibrium constant.

      Sometimes a detail step may differ from problem to problem—it may be more than complex in some problems and less complex in others. Yet, every calculation of equilibrium concentrations from a set of initial concentrations will involve these steps.

      In solving equilibrium bug that involve changes in concentration, sometimes information technology is convenient to set up an ICE tabular array, as described in the previous section.

Example 4

Calculation of Concentration Changes as a Reaction Goes to Equilibrium
Under certain conditions, the equilibrium constant for the decomposition of PCl_five(thou) into PCl₃(g) and Cl₂(thousand) is 0.0211. What are the equilibrium concentrations of PCl₅, PCl₃, and Cl₂ if the initial concentration of PCl₅ was ane.00 M?

Solution
Use the stepwise process described earlier.

1. Determine the direction the reaction proceeds.

   The counterbalanced equation for the decomposition of PCl_v is

   [latex]\text{PCl}_5(chiliad)\;{\rightleftharpoons}\;\text{PCl}_3(g)\;+\;\text{Cl}_2(m)[/latex]

   Because we take no products initially, Q_c = 0 and the reaction volition proceed to the right.

2. Determine the relative changes needed to reach equilibrium, so write the equilibrium concentrations in terms of these changes.

   Let u.s.a. represent the increase in concentration of PCl_iii by the symbol x. The other changes may be written in terms of 10 by because the coefficients in the chemic equation.

   [latex]\begin{array}{lcccc} \text{PCl}_5(g) & {\rightleftharpoons} & \text{PCl}_3(1000) & + & \text{Cl}_2(yard) \\[0.5em] -x & & x & & x \stop{array}[/latex]

   The changes in concentration and the expressions for the equilibrium concentrations are:
   

3. Solve for the change and the equilibrium concentrations.

   Substituting the equilibrium concentrations into the equilibrium abiding equation gives

   [latex]K_c = \frac{[\text{PCl}_3][\text{Cl}_2]}{[\text{PCl}_5]} = 0.0211[/latex]

   [latex]= \frac{(x)(x)}{(1.00\;-\;x)}[/latex]

   This equation contains but one variable, x, the change in concentration. We can write the equation as a quadratic equation and solve for x using the quadratic formula.

   [latex]0.0211 = \frac{(x)(x)}{(1.00\;-\;10)}[/latex]

   [latex]0.0211(ane.00\;-\;10) = 10^2[/latex]

   [latex]x^2\;+\;0.0211x\;-\;0.0211 = 0[/latex]

   Appendix B shows u.s.a. an equation of the form ax ² + bx + c = 0 tin exist rearranged to solve for 10:

   [latex]x = \frac{-b\;{\pm}\;\sqrt{b^2\;-\;4ac}}{2a}[/latex]

   In this case, a = i, b = 0.0211, and c = −0.0211. Substituting the appropriate values for a, b, and c yields:

   [latex]x = \frac{-0.0211\;{\pm}\;\sqrt{(0.0211)^2\;-\;4(one)(-0.0211)}}{2(ane)}[/latex]

   [latex]= \frac{-0.0211\;{\pm}\;\sqrt{(4.45\;\times\;10^{-four})\;+\;(8.44\;\times\;x^{-2})}}{2}[/latex]

   [latex]= \frac{-0.0211\;{\pm}\;0.291}{2}[/latex]

   Hence

   [latex]x = \frac{-0.0211\;+\;0.291}{2} = 0.135[/latex]

   or

   [latex]x = \frac{-0.0211\;-\;0.291}{2} = -0.156[/latex]

   Quadratic equations often have 2 different solutions, one that is physically possible and one that is physically impossible (an extraneous root). In this example, the second solution (−0.156) is physically incommunicable considering we know the change must be a positive number (otherwise we would terminate up with negative values for concentrations of the products). Thus, x = 0.135 M.

   The equilibrium concentrations are

   [latex][\text{PCl}_5] = 1.00\;-\;0.135 = 0.87\;M[/latex]

   [latex][\text{PCl}_3] = x = 0.135\;M[/latex]

   [latex][\text{Cl}_2] = x = 0.135\;Chiliad[/latex]

4. Check the arithmetic.

   Substitution into the expression for G_c (to bank check the adding) gives

   [latex]K_c = \frac{[\text{PCl}_3][\text{Cl}_2]}{[\text{PCl}_5]} = \frac{(0.135)(0.135)}{0.87} = 0.021[/latex]

   The equilibrium constant calculated from the equilibrium concentrations is equal to the value of K_c given in the problem (when rounded to the proper number of significant figures). Thus, the calculated equilibrium concentrations check.

Check Your Learning
Acetic acrid, CH₃CO_iiH, reacts with ethanol, C_twoH₅OH, to form water and ethyl acetate, CH₃CO₂C_iiH₅.

[latex]\text{CH}_3\text{CO}_2\text{H}\;+\;\text{C}_2\text{H}_5\text{OH}\;{\leftrightharpoons}\;\text{CH}_3\text{CO}_2\text{C}_2\text{H}_5\;+\;\text{H}_2\text{O}[/latex]

The equilibrium constant for this reaction with dioxane as a solvent is 4.0. What are the equilibrium concentrations when a mixture that is 0.15 M in CH_threeCO_twoH, 0.15 Grand in C_iiH₅OH, 0.twoscore M in CH₃CO₂C₂H₅, and 0.twoscore M in H_iiO are mixed in enough dioxane to make i.0 50 of solution?

Answer:

[CH₃CO₂H] = 0.36 M, [C₂H_fiveOH] = 0.36 M, [CH₃CO₂C₂H₅] = 0.17 M, [H₂O] = 0.17 Thou

Cheque Your Learning
A one.00-50 flask is filled with 1.00 moles of H_ii and 2.00 moles of I_ii. The value of the equilibrium abiding for the reaction of hydrogen and iodine reacting to form hydrogen iodide is l.5 nether the given conditions. What are the equilibrium concentrations of H₂, I₂, and HI in moles/L?

[latex]\text{H}_2(g)\;+\;\text{I}_2(thousand)\;{\leftrightharpoons}\;two\text{How-do-you-do}(chiliad)[/latex]

Reply:

[H₂] = 0.06 Yard, [I_two] = 1.06 M, [How-do-you-do] = 1.88 M

Sometimes it is possible to utilise chemical insight to find solutions to equilibrium problems without actually solving a quadratic (or more complicated) equation. First, nonetheless, it is useful to verify that equilibrium tin be obtained starting from two extremes: all (or mostly) reactants and all (or mostly) products (similar to what was shown in Figure 2 in Chapter xiii.2 Equilibrium Constants).

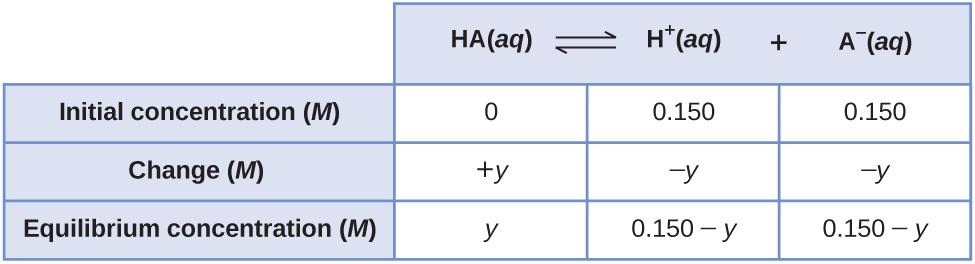
Consider the ionization of 0.150 M HA, a weak acid.

[latex]\text{HA}(aq)\;{\rightleftharpoons}\;\text{H}^{+}(aq)\;+\;\text{A}^{-}(aq)\;\;\;\;\;\;\;K_c = vi.80\;\times\;x^{-four}[/latex]

The most obvious way to determine the equilibrium concentrations would be to start with only reactants. This could be called the "all reactant" starting signal. Using x for the corporeality of acid ionized at equilibrium, this is the Ice table and solution.

Setting up and solving the quadratic equation gives

[latex]K_c = \frac{[\text{H}^{+}][\text{A}^{-}]}{[\text{HA}]} = \frac{(x)(x)}{(0.150\;-\;x)} = half-dozen.80\;\times\;10^{-4}[/latex]

[latex]10^2\;+\;(6.80\;\times\;10^{-4}x)\;-\;(1.02\;\times\;10^{-4}) = 0[/latex]

[latex]x = \frac{-vi.80\;\times\;10^{-iv}\;{\pm}\;\sqrt{(vi.80\;\times\;x^{-4})^2\;-\;(4)(ane)(-1.02\;\times\;10^{-four})}}{(ii)(one)}[/latex]

[latex]ten = 0.00977\;M\;\text{or}\;-0.0104\;Yard[/latex]

Using the positive (physical) root, the equilibrium concentrations are

[latex][\text{HA}] = 0.150\;-\;x = 0.140\;Thousand[/latex]

[latex][\text{H}^{+}] = [\text{A}^{-}] = x = 0.00977\;M[/latex]

A less obvious way to solve the problem would exist to assume all the HA ionizes first, then the system comes to equilibrium. This could exist chosen the "all product" starting bespeak. Assuming all of the HA ionizes gives

[latex][\text{HA}] = 0.150\;-\;0.150 = 0\;M[/latex]

[latex][\text{H}^{+}] = 0\;+\;0.150 = 0.150\;M[/latex]

[latex][\text{A}^{-}] = 0\;+\;0.150 = 0.150\;M[/latex]

Using these every bit initial concentrations and "y" to correspond the concentration of HA at equilibrium, this is the Ice table for this starting point.

Setting up and solving the quadratic equation gives

[latex]K_c = \frac{[\text{H}^{+}][\text{A}^{-}]}{[\text{HA}]} = \frac{(0.150\;-\;y)(0.150\;-\;y)}{(y)} = 6.80\;\times\;10^{-4}[/latex]

[latex]6.80\;\times\;10^{-4}y = 0.0225\;-\;0.300y\;+\;y^2[/latex]

Retain a few extra significant figures to minimize rounding bug.

[latex]y^2\;-\;0.30068y\;+\;0.022500 = 0[/latex]

[latex]y = \frac{0.30068\;{\pm}\;\sqrt{(0.30068)^2\;-\;(iv)(one)(0.022500)}}{(two)(i)}[/latex]

[latex]y = \frac{0.30068\;{\pm}\;0.020210}{two}[/latex]

Rounding each solution to three meaning figures gives

[latex]y = 0.160\;M\;\;\;\;\;\;\;\text{or}\;\;\;\;\;\;\;y = 0.140\;M[/latex]

Using the physically significant root (0.140 G) gives the equilibrium concentrations as

[latex][\text{HA}] = y = 0.140\;One thousand[/latex]

[latex][\text{H}^{+}] = 0.150\;-\;y = 0.010\;M[/latex]

[latex][\text{A}^{-}] = 0.150\;-\;y = 0.010\;G[/latex]

Thus, the two approaches give the same results (to three decimal places), and show that both starting points pb to the same equilibrium conditions. The "all reactant" starting point resulted in a relatively small change (ten) because the system was close to equilibrium, while the "all product" starting point had a relatively large change (y) that was nearly the size of the initial concentrations. It tin can exist said that a organization that starts "close" to equilibrium will crave only a "small" modify in conditions (ten) to reach equilibrium.

Call up that a small Chiliad_c means that very little of the reactants grade products and a large K_c means that about of the reactants class products. If the arrangement tin can exist bundled then it starts "close" to equilibrium, then if the modify (x) is small compared to whatever initial concentrations, it can be neglected. Small-scale is usually defined as resulting in an mistake of less than 5%. The post-obit two examples demonstrate this.

Case 5

Approximate Solution Starting Close to Equilibrium
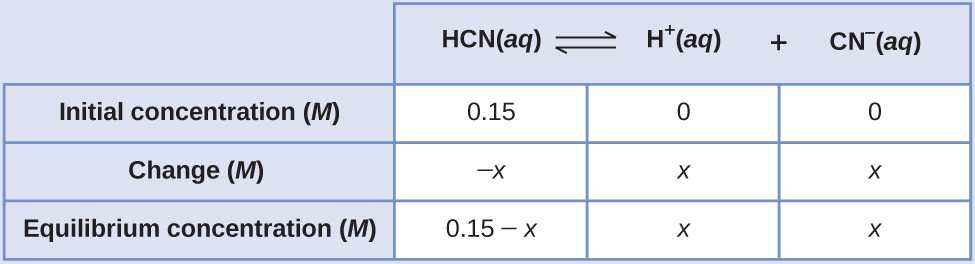
What are the concentrations at equilibrium of a 0.15 M solution of HCN?

[latex]\text{HCN}(aq)\;{\rightleftharpoons}\;\text{H}^{+}(aq)\;+\;\text{CN}^{-}(aq)\;\;\;\;\;\;\;K_c = iv.nine\;\times\;10^{-10}[/latex]

Solution
Using "x" to stand for the concentration of each product at equilibrium gives this Water ice table.

The exact solution may be obtained using the quadratic formula with

[latex]K_c = \frac{(x)(10)}{0.fifteen\;-\;10}[/latex]

solving

[latex]10^2\;+\;four.nine\;\times\;10^{-ten}\;-\;vii.35\;\times\;10^{-11} = 0[/latex]

[latex]x = 8.56\;\times\;10^{-6}\;M\;(iii\;\text{sig.\;figs.}) = 8.half dozen\;\times\;10^{-6}\;G\;(ii\;\text{sig.\;figs.})[/latex]

Thus [H⁺] = [CN^–] = x = 8.6 × 10^–6 One thousand and [HCN] = 0.xv – x = 0.15 Yard.

In this case, chemic intuition tin provide a simpler solution. From the equilibrium constant and the initial weather condition, x must exist small compared to 0.xv Grand. More formally, if [latex]x\;{\ll}\;0.15[/latex], and so 0.15 – x ≈ 0.15. If this assumption is truthful, then information technology simplifies obtaining x

[latex]K_c = \frac{(x)(x)}{0.15\;-\;10}\;{\approx}\;\frac{x^2}{0.15}[/latex]

[latex]4.9\;\times\;10^{-x} = \frac{x^two}{0.fifteen}[/latex]

[latex]x^two = (0.15)(iv.9\;\times\;10^{-10}) = seven.4\;\times\;10^{-11}[/latex]

[latex]10 = \sqrt{7.4\;\times\;x^{-eleven}} = eight.6\;\times\;10^{-6}\;M[/latex]

In this example, solving the exact (quadratic) equation and using approximations gave the same result to two meaning figures. While near of the time the approximation is a chip different from the exact solution, equally long as the error is less than v%, the approximate solution is considered valid. In this problem, the 5% applies to IF (0.fifteen – x) ≈ 0.15 M, so if

[latex]\frac{x}{0.15}\;\times\;100\% = \frac{8.6\;\times\;10^{-6}}{0.fifteen}\;\times\;100\% = 0.006\%[/latex]

is less than five%, every bit it is in this instance, the supposition is valid. The approximate solution is thus a valid solution.

Check Your Learning
What are the equilibrium concentrations in a 0.25 G NH₃ solution?

[latex]\text{NH}_3(aq)\;+\;\text{H}_2\text{O}(l)\;{\rightleftharpoons}\;\text{NH}_4^{\;\;+}(aq)\;+\;\text{OH}^{-}(aq)\;\;\;\;\;\;\;K_c = 1.8\;\times\;ten^{-v}[/latex]

Assume that x is much less than 0.25 M and summate the mistake in your assumption.

Answer:

[latex][\text{OH}^{-}] = [\text{NH}_4^{\;\;+}] = 0.0021\;Thousand[/latex]; [NH₃] = 0.25 K, fault = 0.84%

The second example requires that the original information be processed a flake, only it still can be solved using a small x approximation.

Example 6

Estimate Solution Afterwards Shifting Starting Concentration
Copper(2) ions course a complex ion in the presence of ammonia

[latex]\text{Cu}^{2+}(aq)\;+\;4\text{NH}_3(aq)\;{\rightleftharpoons}\;\text{Cu(NH}_3)_4^{\;\;2+}(aq)\;\;\;\;\;\;\;K_c = 5.0\;\times\;10^{thirteen} = \frac{[\text{Cu(NH}_3)_4^{\;\;2+}]}{[\text{Cu}^{2+}(aq)][\text{NH}_3]^4}[/latex]

If 0.010 mol Cu²⁺ is added to 1.00 50 of a solution that is one.00 M NH₃ what are the concentrations when the system comes to equilibrium?

Solution
The initial concentration of copper(II) is 0.010 Yard. The equilibrium constant is very big and then it would exist meliorate to start with as much production every bit possible because "all products" is much closer to equilibrium than "all reactants." Note that Cu²⁺ is the limiting reactant; if all 0.010 Yard of it reacts to course product the concentrations would exist

[latex][\text{Cu}^{2+}] = 0.010\;-\;0.010 = 0\;M[/latex]

[latex][\text{Cu(NH}_3)_4^{\;\;two+}] = 0.010\;Yard[/latex]

[latex][\text{NH}_3] = ane.00\;-\;4\;\times\;0.010 = 0.96\;M[/latex]

Using these "shifted" values as initial concentrations with x equally the complimentary copper(2) ion concentration at equilibrium gives this Water ice table.

Since we are starting close to equilibrium, x should be pocket-sized then that

[latex]0.96\;+\;4x\;{\approx}\;0.96\;K[/latex]

[latex]0.010\;-\;10\;{\approx}\;0.010\;G[/latex]

[latex]K_c = \frac{(0.010\;-\;ten)}{x(0.96\;-\;4x)^iv}\;{\approx}\;\frac{(0.010)}{10(0.96)^4} = 5.0\;\times\;10^{13}[/latex]

[latex]x = \frac{(0.010)}{K_c(0.96)^iv} = 2.four\;\times\;10^{-16}\;K[/latex]

Select the smallest concentration for the 5% dominion.

[latex]\frac{ii.4\;\times\;10^{-16}}{0.010}\;\times\;100\% = ii\;\times\;10^{-12}\%[/latex]

This is much less than 5%, so the assumptions are valid. The concentrations at equilibrium are

[latex][\text{Cu}^{2+}] = x = 2.four\;\times\;x^{-16}\;M[/latex]

[latex][\text{NH}_3] = 0.96\;-\;4x = 0.96\;M[/latex]

[latex][\text{Cu(NH}_3)_4^{\;\;ii+}] = 0.010\;-\;x = 0.010\;K[/latex]

By starting with the maximum amount of product, this system was near equilibrium and the change (ten) was very small. With merely a small change required to get to equilibrium, the equation for x was greatly simplified and gave a valid result well within the 5% mistake maximum.

Check Your Learning
What are the equilibrium concentrations when 0.25 mol Ni²⁺ is added to 1.00 L of 2.00 M NH₃ solution?

[latex]\text{Ni}^{ii+}(aq)\;+\;6\text{NH}_3(aq)\;{\rightleftharpoons}\;\text{Ni(NH}_3)_6^{\;\;2+}(aq)\;\;\;\;\;\;\;K_c = five.five\;\times\;10^eight[/latex]

With such a big equilibrium abiding, outset class as much product as possible, then assume that but a pocket-sized amount (x) of the product shifts left. Calculate the error in your assumption.

Reply:

[latex][\text{Ni(NH}_3)_6^{\;\;two+}] = 0.25\;Thou[/latex], [NH₃] = 0.50 Thou, [Ni²⁺] = 2.9 × 10^–8 M, error = 1.2 × x^–5%

Key Concepts and Summary

The ratios of the rate of change in concentrations of a reaction are equal to the ratios of the coefficients in the balanced chemical equation. The sign of the coefficient of X is positive when the concentration increases and negative when it decreases. We learned to approach three bones types of equilibrium bug. When given the concentrations of the reactants and products at equilibrium, nosotros tin solve for the equilibrium constant; when given the equilibrium constant and some of the concentrations involved, we tin can solve for the missing concentrations; and when given the equilibrium constant and the initial concentrations, nosotros can solve for the concentrations at equilibrium.

Chemistry End of Affiliate Exercises

1. A reaction is represented past this equation: [latex]\text{A}(aq)\;+\;two\text{B}(aq)\;{\rightleftharpoons}\;2\text{C}(aq)\;\;\;\;\;\;\;K_c = 1\;\times\;10^3[/latex]

   (a) Write the mathematical expression for the equilibrium abiding.

   (b) Using concentrations ≤1 M, make upwards 2 sets of concentrations that describe a mixture of A, B, and C at equilibrium.

2. A reaction is represented by this equation: [latex]ii\text{W}(aq)\;{\rightleftharpoons}\;\text{Ten}(aq)\;+\;2\text{Y}(aq)\;\;\;\;\;\;\;K_c = 5\;\times\;10^{-4}[/latex]

   (a) Write the mathematical expression for the equilibrium constant.

   (b) Using concentrations of ≤1 M, brand upwards two sets of concentrations that describe a mixture of Due west, X, and Y at equilibrium.

3. What is the value of the equilibrium constant at 500 °C for the formation of NH_three according to the post-obit equation?

   [latex]\text{N}_2(g)\;+\;3\text{H}_2(g)\;{\rightleftharpoons}\;2\text{NH}_3(1000)[/latex]

   An equilibrium mixture of NH_three(g), H₂(thousand), and Due north₂(g) at 500 °C was found to contain 1.35 Thousand H₂, 1.xv M N₂, and 4.12 × x^−ane M NH₃.

4. Hydrogen is prepared commercially past the reaction of marsh gas and h2o vapor at elevated temperatures.

   [latex]\text{CH}_4(1000)\;+\;\text{H}_2\text{O}(g)\;{\rightleftharpoons}\;3\text{H}_2(m)\;+\;\text{CO}(g)[/latex]

   What is the equilibrium constant for the reaction if a mixture at equilibrium contains gases with the post-obit concentrations: CH₄, 0.126 Yard; H₂O, 0.242 K; CO, 0.126 One thousand; H₂ 1.fifteen M, at a temperature of 760 °C?

5. A 0.72-mol sample of PCl_five is put into a one.00-L vessel and heated. At equilibrium, the vessel contains 0.40 mol of PCl₃(g) and 0.40 mol of Cl₂(g). Calculate the value of the equilibrium constant for the decomposition of PCl₅ to PCl₃ and Cl₂ at this temperature.
6. At 1 atm and 25 °C, NO₂ with an initial concentration of 1.00 M is 3.3 × 10⁻³% decomposed into NO and O₂. Calculate the value of the equilibrium abiding for the reaction.

   [latex]2\text{NO}_2(g)\;{\rightleftharpoons}\;2\text{NO}(one thousand)\;+\;\text{O}_2(grand)[/latex]

7. Calculate the value of the equilibrium constant One thousand_P for the reaction [latex]2\text{NO}(one thousand)\;+\;\text{Cl}_2(g)\;{\rightleftharpoons}\;2\text{NOCl}(1000)[/latex] from these equilibrium pressures: NO, 0.050 atm; Cl_two, 0.xxx atm; NOCl, 1.2 atm.
8. When heated, iodine vapor dissociates according to this equation:

   [latex]\text{I}_2(1000)\;{\rightleftharpoons}\;2\text{I}(g)[/latex]

   At 1274 K, a sample exhibits a partial pressure of I₂ of 0.1122 atm and a partial pressure due to I atoms of 0.1378 atm. Make up one's mind the value of the equilibrium constant, Thousand_P , for the decomposition at 1274 K.

9. A sample of ammonium chloride was heated in a closed container.

   [latex]\text{NH}_4\text{Cl}(southward)\;{\rightleftharpoons}\;\text{NH}_3(one thousand)\;+\;\text{HCl}(chiliad)[/latex]

   At equilibrium, the force per unit area of NH₃(1000) was establish to be i.75 atm. What is the value of the equilibrium constant Thousand_P for the decomposition at this temperature?

10. At a temperature of 60 °C, the vapor pressure level of water is 0.196 atm. What is the value of the equilibrium constant One thousand_P for the transformation at 60 °C?

    [latex]\text{H}_2\text{O}(l)\;{\rightleftharpoons}\;\text{H}_2\text{O}(g)[/latex]

11. Complete the changes in concentrations (or force per unit area, if requested) for each of the post-obit reactions.

    (a)

    [latex]\begin{array}{lcccc} 2\text{SO}_3(chiliad) & {\rightleftharpoons} & 2\text{SO}_2(k) & + & \text{O}_2(g) \\[0.5em] \rule[0ex]{ii.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & +10 \\[0.5em] \dominion[0ex]{2.5em}{0.1ex} & & \rule[0ex]{two.5em}{0.1ex} & & 0.125\;One thousand \end{assortment}[/latex]

    (b)

    [latex]\begin{assortment}{lcccccc} iv\text{NH}_3(g) & + & 3\text{O}_2(g) & {\rightleftharpoons} & ii\text{N}_2(g) & + & six\text{H}_2\text{O}(m) \\[0.5em] \rule[0ex]{ii.5em}{0.1ex} & & 3x & & \rule[0ex]{2.5em}{0.1ex} & & \dominion[0ex]{2.5em}{0.1ex} \\[0.5em] \rule[0ex]{2.5em}{0.1ex} & & 0.24\;One thousand & & \dominion[0ex]{two.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} \terminate{array}[/latex]

    (c) Change in pressure level:

    [latex]\brainstorm{array}{lcccc} 2\text{CH}_4(thousand) & {\rightleftharpoons} & \text{C}_2\text{H}_2(thousand) & + & 3\text{H}_2(thou) \\[0.5em] \rule[0ex]{ii.5em}{0.1ex} & & x & & \dominion[0ex]{2.5em}{0.1ex} \\[0.5em] \rule[0ex]{2.5em}{0.1ex} & & 25\;\text{torr} & & \rule[0ex]{2.5em}{0.1ex} \finish{array}[/latex]

    (d) Change in force per unit area:

    [latex]\begin{array}{lcccccc} \text{CH}_4(chiliad) & + & \text{H}_2\text{O}(grand) & {\rightleftharpoons} & \text{CO}(1000) & + & iii\text{H}_2(g) \\[0.5em] \rule[0ex]{2.5em}{0.1ex} & & ten & & \dominion[0ex]{2.5em}{0.1ex} & & \dominion[0ex]{two.5em}{0.1ex} \\[0.5em] \dominion[0ex]{two.5em}{0.1ex} & & 5\;\text{atm} & & \rule[0ex]{ii.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} \finish{array}[/latex]

    (e)

    [latex]\brainstorm{assortment}{lcccc} \text{NH}_4\text{Cl}(south) & {\rightleftharpoons} & \text{NH}_3(g) & + & \text{HCl}(g) \\[0.5em] & & x & & \dominion[0ex]{2.5em}{0.1ex} \\[0.5em] & & one.03\;\times\;10^{-4}\;Yard & & \dominion[0ex]{two.5em}{0.1ex} \end{array}[/latex]

    (f) change in force per unit area:

    [latex]\brainstorm{assortment}{lcccc} \text{Ni}(s) & + & 4\text{CO}(g) & {\leftrightharpoons} & \text{Ni(CO)}_4(k) \\[0.5em] & & 4x & & \rule[0ex]{2.5em}{0.1ex} \\[0.5em] & & 0.40\;\text{atm} & & \rule[0ex]{two.5em}{0.1ex} \end{array}[/latex]

12. Complete the changes in concentrations (or pressure, if requested) for each of the following reactions.

    (a)

    [latex]\begin{array}{lcccc} 2\text{H}_2(g) & + & \text{O}_2(g) & {\rightleftharpoons} & 2\text{H}_2\text{O}(one thousand) \\[0.5em] \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & &+2x \\[0.5em] \dominion[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & ane.50\;M \cease{array}[/latex]

    (b)

    [latex]\begin{assortment}{lcccccc} \text{CS}_2(m) & + & four\text{H}_2(g) & {\rightleftharpoons} & \text{CH}_4(g) & + & 2\text{H}_2\text{Due south}(grand) \\[0.5em] 10 & & \rule[0ex]{2.5em}{0.1ex} & & \dominion[0ex]{ii.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} \\[0.5em] 0.020\;Thou & & \dominion[0ex]{ii.5em}{0.1ex} & & \rule[0ex]{two.5em}{0.1ex} & & \dominion[0ex]{2.5em}{0.1ex} \end{array}[/latex]

    (c) Modify in pressure level:

    [latex]\begin{array}{lcccc} \text{H}_2(g) & + & \text{Cl}_2(thousand) & {\rightleftharpoons} & two\text{HCl}(grand) \\[0.5em] x & & \rule[0ex]{ii.5em}{0.1ex} & & \dominion[0ex]{2.5em}{0.1ex} \\[0.5em] 1.50\;\text{atm} & & \dominion[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} \stop{array}[/latex]

    (d) Change in pressure:

    [latex]\begin{array}{lcccccc} 2\text{NH}_3(g) & + & 2\text{O}_2(g) & {\rightleftharpoons} & \text{Northward}_2\text{O}(g) & + & iii\text{H}_2\text{O}(g) \\[0.5em] \rule[0ex]{ii.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{two.5em}{0.1ex} & & 10 \\[0.5em] \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & \rule[0ex]{2.5em}{0.1ex} & & 60.6\;\text{torr} \cease{array}[/latex]

    (east)

    [latex]\brainstorm{array}{lcccc} \text{NH}_4\text{HS}(s) & {\leftrightharpoons} & \text{NH}_3(g) & + & \text{H}_2\text{S}(thousand) \\[0.5em] & & x & & \rule[0ex]{2.5em}{0.1ex} \\[0.5em] & & 9.8\;\times\;x^{-half dozen}\;Thousand & & \rule[0ex]{2.5em}{0.1ex} \cease{array}[/latex]

    (f) Alter in pressure:

    [latex]\begin{array}{lcccc} \text{Fe}(south) & + & 5\text{CO}(thousand) & {\leftrightharpoons} & \text{Fe(CO)}_4(m) \\[0.5em] & & \rule[0ex]{two.5em}{0.1ex} & & x \\[0.5em] & & \dominion[0ex]{2.5em}{0.1ex} & & 0.012\;\text{atm} \end{array}[/latex]

13. Why are at that place no changes specified for Ni in Exercise 11, part (f)? What property of Ni does change?
14. Why are there no changes specified for NH₄HS in Exercise 12, part (e)? What property of NH₄HS does change?
15. Analysis of the gases in a sealed reaction vessel containing NH₃, North_ii, and H₂ at equilibrium at 400 °C established the concentration of N₂ to be 1.two Yard and the concentration of H₂ to be 0.24 1000.

    [latex]\text{Due north}_2(m)\;+\;iii\text{H}_2(1000)\;{\rightleftharpoons}\;2\text{NH}_3(thousand)\;\;\;\;\;\;\;K_c = 0.50\;\text{at}\;400\;^{\circ}\text{C}[/latex]

    Calculate the equilibrium molar concentration of NH₃.

16. Calculate the number of moles of HI that are at equilibrium with ane.25 mol of H₂ and 1.25 mol of I_ii in a five.00−Fifty flask at 448 °C.

    [latex]\text{H}_2\;+\;\text{I}_2\;{\rightleftharpoons}\;ii\text{Hullo}\;\;\;\;\;\;\;K_c = 50.2\;\text{at}\;448\;^{\circ}\text{C}[/latex]

17. What is the pressure of BrCl in an equilibrium mixture of Cl₂, Br_ii, and BrCl if the pressure of Cl₂ in the mixture is 0.115 atm and the force per unit area of Br_two in the mixture is 0.450 atm?

    [latex]\text{Cl}_2(g)\;+\;\text{Br}_2(grand)\;{\rightleftharpoons}\;2\text{BrCl}(g)\;\;\;\;\;\;\;K_P = 4.vii\;\times\;ten^{-2}[/latex]

18. What is the force per unit area of CO₂ in a mixture at equilibrium that contains 0.50 atm H₂, ii.0 atm of H₂O, and 1.0 atm of CO at 990 °C?

    [latex]\text{H}_2(k)\;+\;\text{CO}_2(m)\;{\rightleftharpoons}\;\text{H}_2\text{O}(thousand)\;+\;\text{CO}(chiliad)\;\;\;\;\;\;\;K_P = ane.6\;\text{at}\;990\;^{\circ}\text{C}[/latex]

19. Cobalt metal can be prepared by reducing cobalt(Two) oxide with carbon monoxide.

    [latex]\text{CoO}(s)\;+\;\text{CO}(g)\;{\rightleftharpoons}\;\text{Co}(due south)\;+\;\text{CO}_2(one thousand)\;\;\;\;\;\;\;K_c = iv.90\;\times\;10^two\;\text{at}\;550\;^{\circ}\text{C}[/latex]

    What concentration of CO remains in an equilibrium mixture with [CO₂] = 0.100 M?

20. Carbon reacts with water vapor at elevated temperatures.

    [latex]\text{C}(southward)\;+\;\text{H}_2\text{O}(g)\;{\rightleftharpoons}\;\text{CO}(g)\;+\;\text{H}_2(g)\;\;\;\;\;\;\;K_c = 0.2\;\text{at}\;chiliad\;^{\circ}\text{C}[/latex]

    What is the concentration of CO in an equilibrium mixture with [H_iiO] = 0.500 G at 1000 °C?

21. Sodium sulfate 10−hydrate, Na₂So₄·10H_iiO, dehydrates according to the equation

    [latex]\text{Na}_2\text{SO}_4{\cdot}x\text{H}_2\text{O}(s)\;{\rightleftharpoons}\;\text{Na}_2\text{SO}_4(s)\;+\;10\text{H}_2\text{O}(g)\;\;\;\;\;\;\;K_P = 4.08\;\times\;10^{-25}\;\text{at}\;25\;^{\circ}\text{C}[/latex]

    What is the pressure of water vapor at equilibrium with a mixture of Na₂SO_four·10H₂O and NaSO_four?

22. Calcium chloride 6−hydrate, CaCl₂·6H₂O, dehydrates according to the equation

    [latex]\text{CaCl}_2{\cdot}6\text{H}_2\text{O}(s)\;{\rightleftharpoons}\;\text{CaCl}_2(s)\;+\;6\text{H}_2\text{O}(yard)\;\;\;\;\;\;\;K_P = v.09\;\times\;10^{-44}\;\text{at}\;25\;^{\circ}\text{C}[/latex]

    What is the pressure of h2o vapor at equilibrium with a mixture of CaCl₂·6H₂O and CaCl_two?

23. A student solved the following problem and found the equilibrium concentrations to be [Then_two] = 0.590 Thousand, [O_ii] = 0.0450 G, and [Then₃] = 0.260 Grand. How could this student cheque the work without reworking the trouble? The problem was: For the post-obit reaction at 600 °C:

    [latex]2\text{Then}_2(thousand)\;+\;\text{O}_2(g)\;{\rightleftharpoons}\;2\text{And then}_3(thousand)\;\;\;\;\;\;\;K_c = 4.32[/latex]

    What are the equilibrium concentrations of all species in a mixture that was prepared with [And then_iii] = 0.500 M, [SO_two] = 0 1000, and [O₂] = 0.350 M?

24. A student solved the following trouble and establish [N_iiO₄] = 0.16 M at equilibrium. How could this student recognize that the reply was wrong without reworking the trouble? The problem was: What is the equilibrium concentration of Northward_twoO₄ in a mixture formed from a sample of NO₂ with a concentration of 0.10 M?

    [latex]2\text{NO}_2(one thousand)\;{\rightleftharpoons}\;\text{Due north}_2\text{O}_4(g)\;\;\;\;\;\;\;K_c = 160[/latex]

25. Presume that the change in concentration of North₂O_four is minor enough to be neglected in the following problem.
    (a) Calculate the equilibrium concentration of both species in 1.00 L of a solution prepared from 0.129 mol of N_twoO₄ with chloroform as the solvent.

    [latex]\text{N}_2\text{O}_4(grand)\;{\leftrightharpoons}\;2\text{NO}_2(g)\;\;\;\;\;\;\;K_c = 1.07\;\times\;10^{-v}[/latex] in chloroform

    (b) Show that the alter is small enough to be neglected.

26. Assume that the modify in concentration of COCl₂ is modest enough to be neglected in the following problem.

    (a) Calculate the equilibrium concentration of all species in an equilibrium mixture that results from the decomposition of COCl₂ with an initial concentration of 0.3166 Thou.

    [latex]\text{COCl}_2(g)\;{\rightleftharpoons}\;\text{CO}(g)\;+\;\text{Cl}_2(g)\;\;\;\;\;\;\;K_c = two.2\;\times\;ten^{-10}[/latex]

    (b) Testify that the change is small plenty to be neglected.

27. Assume that the change in pressure of H₂S is small enough to exist neglected in the following trouble.

    (a) Calculate the equilibrium pressures of all species in an equilibrium mixture that results from the decomposition of H₂S with an initial pressure of 0.824 atm.

    [latex]2\text{H}_2\text{Due south}(g)\;{\rightleftharpoons}\;ii\text{H}_2(g)\;+\;\text{Southward}_2(thousand)\;\;\;\;\;\;\;K_P = 2.two\;\times\;x^{-6}[/latex]

    (b) Prove that the alter is pocket-size enough to be neglected.

28. What are all concentrations after a mixture that contains [H₂O] = ane.00 M and [Cl_iiO] = one.00 Thou comes to equilibrium at 25 °C?

    [latex]\text{H}_2\text{O}(m)\;+\;\text{Cl}_2\text{O}(g)\;{\rightleftharpoons}\;2\text{HOCl}(yard)\;\;\;\;\;\;\;K_c = 0.0900[/latex]

29. What are the concentrations of PCl_v, PCl_three, and Cl₂ in an equilibrium mixture produced by the decomposition of a sample of pure PCl₅ with [PCl₅] = ii.00 M?

    [latex]\text{PCl}_5(g)\;{\rightleftharpoons}\;\text{PCl}_3(g)\;+\;\text{Cl}_2(m)\;\;\;\;\;\;\;K_c = 0.0211[/latex]

30. Calculate the pressures of all species at equilibrium in a mixture of NOCl, NO, and Cl₂ produced when a sample of NOCl with a pressure of 10.0 atm comes to equilibrium co-ordinate to this reaction:
    [latex]2\text{NOCl}(g)\;{\rightleftharpoons}\;two\text{NO}(g)\;+\;\text{Cl}_2(grand)\;\;\;\;\;\;\;K_P = 4.0\;\times\;10^{-4}[/latex]
31. Summate the equilibrium concentrations of NO, O₂, and NO₂ in a mixture at 250 °C that results from the reaction of 0.20 1000 NO and 0.ten M O_two. (Hint: Thousand is large; presume the reaction goes to completion then comes back to equilibrium.)

    [latex]2\text{NO}(thou)\;+\;\text{O}_2(chiliad)\;{\rightleftharpoons}\;2\text{NO}_2(g)\;\;\;\;\;\;\;K_c = 2.three\;\times\;10^v\;\text{at}\;250\;^{\circ}\text{C}[/latex]

32. Summate the equilibrium concentrations that result when 0.25 M O_ii and ane.0 Yard HCl react and come to equilibrium.

    [latex]iv\text{HCl}(k)\;+\;\text{O}_2(g)\;{\rightleftharpoons}\;2\text{Cl}_2(thou)\;+\;2\text{H}_2\text{O}(g)\;\;\;\;\;\;\;K_c = 3.1\;\times\;10^{13}[/latex]

33. One of the important reactions in the formation of smog is represented by the equation

    [latex]\text{O}_3(g)\;+\;\text{NO}(g)\;{\rightleftharpoons}\;\text{NO}_2(chiliad)\;+\;\text{O}_2(g)\;\;\;\;\;\;\;K_P = 6.0\;\times\;10^{34}[/latex]

    What is the force per unit area of O₃ remaining later on a mixture of O₃ with a pressure of ane.2 × ten⁻⁸ atm and NO with a pressure level of 1.2 × x⁻⁸ atm comes to equilibrium? (Hint: M_P is large; presume the reaction goes to completion then comes back to equilibrium.)

34. Calculate the pressures of NO, Cl₂, and NOCl in an equilibrium mixture produced by the reaction of a starting mixture with iv.0 atm NO and 2.0 atm Cl₂. (Hint: Thousand_P is modest; assume the opposite reaction goes to completion and so comes dorsum to equilibrium.)

    [latex]ii\text{NO}(g)\;+\;\text{Cl}_2(one thousand)\;{\rightleftharpoons}\;2\text{NOCl}(k)\;\;\;\;\;\;\;K_P = two.v\;\times\;x^iii[/latex]

35. Calculate the number of grams of HI that are at equilibrium with 1.25 mol of H₂ and 63.5 g of iodine at 448 °C.

    [latex]\text{H}_2\;+\;\text{I}_2\;{\rightleftharpoons}\;2\text{Hullo}\;\;\;\;\;\;\;K_c = fifty.two\;\text{at}\;448\;^{\circ}\text{C}[/latex]

36. Butane exists as two isomers, n−butane and isobutane.
    

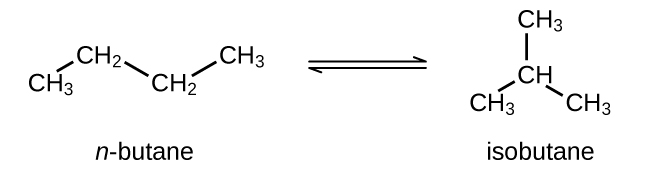
    Yard_P = 2.5 at 25 °C

    What is the pressure of isobutane in a container of the two isomers at equilibrium with a full pressure level of 1.22 atm?

37. What is the minimum mass of CaCO₃ required to establish equilibrium at a certain temperature in a half dozen.50-L container if the equilibrium constant (K_c ) is 0.050 for the decomposition reaction of CaCO₃ at that temperature?

    [latex]\text{CaCO}_3(due south)\;{\rightleftharpoons}\;\text{CaO}(s)\;+\;\text{CO}_2(grand)[/latex]

38. The equilibrium constant (K_c ) for this reaction is 1.60 at 990 °C:

    [latex]\text{H}_2(g)\;+\;\text{CO}_2(g)\;{\rightleftharpoons}\;\text{H}_2\text{O}(g)\;+\;\text{CO}(m)[/latex]

    Calculate the number of moles of each component in the final equilibrium mixture obtained from adding ane.00 mol of H_ii, 2.00 mol of CO_two, 0.750 mol of H₂O, and 1.00 mol of CO to a five.00-L container at 990 °C.

39. At 25 °C and at 1 atm, the partial pressures in an equilibrium mixture of N₂O₄ and NO_two are [latex]\text{P}_{\text{N}_2\text{O}_4} = 0.lxx\;\text{atm}[/latex] and [latex]\text{P}_{\text{NO}_2} = 0.thirty\;\text{atm}[/latex].

    (a) Predict how the pressures of NO₂ and Northward₂O₄ will change if the total pressure increases to ix.0 atm. Will they increment, subtract, or remain the same?

    (b) Calculate the partial pressures of NO_two and Due north₂O_four when they are at equilibrium at nine.0 atm and 25 °C.

40. In a three.0-L vessel, the following equilibrium partial pressures are measured: Due north₂, 190 torr; H₂, 317 torr; NH₃, i.00 × ten³ torr.

    [latex]\text{N}_2(g)\;+\;3\text{H}_2(g)\;{\rightleftharpoons}\;2\text{NH}_3(k)[/latex]

    (a) How will the partial pressures of H_ii, N₂, and NH_three change if H_ii is removed from the organization? Will they increment, subtract, or remain the same?

    (b) Hydrogen is removed from the vessel until the partial pressure of nitrogen, at equilibrium, is 250 torr. Calculate the fractional pressures of the other substances under the new conditions.

41. The equilibrium constant (G_c ) for this reaction is five.0 at a given temperature.

    [latex]\text{CO}(g)\;+\;\text{H}_2\text{O}(g)\;{\rightleftharpoons}\;\text{CO}_2(chiliad)\;+\;\text{H}_2(g)[/latex]

    (a) On analysis, an equilibrium mixture of the substances present at the given temperature was found to incorporate 0.xx mol of CO, 0.thirty mol of water vapor, and 0.ninety mol of H₂ in a liter. How many moles of CO₂ were there in the equilibrium mixture?

    (b) Maintaining the same temperature, additional H_two was added to the organization, and some water vapor was removed by drying. A new equilibrium mixture was thereby established containing 0.twoscore mol of CO, 0.30 mol of water vapor, and 1.ii mol of H₂ in a liter. How many moles of CO_ii were in the new equilibrium mixture? Compare this with the quantity in part (a), and talk over whether the second value is reasonable. Explicate how it is possible for the h2o vapor concentration to be the same in the two equilibrium solutions even though some vapor was removed before the second equilibrium was established.

42. Antimony pentachloride decomposes co-ordinate to this equation:

    [latex]\text{SbCl}_5(thou)\;{\rightleftharpoons}\;\text{SbCl}_3(g)\;+\;\text{Cl}_2(k)[/latex]

    An equilibrium mixture in a 5.00-L flask at 448 °C contains 3.85 g of SbCl₅, 9.14 g of SbCl_three, and ii.84 g of Cl₂. How many grams of each will exist found if the mixture is transferred into a two.00-L flask at the same temperature?

43. Consider the reaction between H_two and O_ii at 1000 K
    [latex]2\text{H}_2(chiliad)\;+\;\text{O}_2(g)\;{\rightleftharpoons}\;ii\text{H}_2\text{O}(g)\;\;\;\;\;\;\;K_P = \frac{(P_{\text{H}_2\text{O}})^2}{(P_{\text{O}_2})(P_{\text{H}_2})^3} = one.33\;\times\;10^{20}[/latex]

    If 0.500 atm of H_ii and 0.500 atm of O_two are immune to come to equilibrium at this temperature, what are the fractional pressures of the components?

44. An equilibrium is established according to the following equation

    [latex]\text{Hg}_2^{\;\;2+}(aq)\;+\;\text{NO}_3^{\;\;-}(aq)\;+\;three\text{H}^{+}(aq)\;{\rightleftharpoons}\;2\text{Hg}^{2+}(aq)\;+\;\text{HNO}_2(aq)\;+\;\text{H}_2\text{O}(l)\;\;\;\;\;\;\;K_c = iv.half dozen[/latex]

    What will happen in a solution that is 0.20 K each in [latex]\text{Hg}_2^{\;\;ii+}[/latex], [latex]\text{NO}_3^{\;\;-}[/latex], H⁺, Hg²⁺, and HNO₂?

    (a) [latex]\text{Hg}_2^{\;\;2+}[/latex] volition be oxidized and [latex]\text{NO}_3^{\;\;-}[/latex] reduced.

    (b) [latex]\text{Hg}_2^{\;\;2+}[/latex] volition exist reduced and [latex]\text{NO}_3^{\;\;-}[/latex] oxidized.

    (c) Hg²⁺ volition be oxidized and HNO_ii reduced.

    (d) Hg²⁺ will be reduced and HNO₂ oxidized.

    (e) There will exist no modify because all reactants and products accept an activity of one.

45. Consider the equilibrium

    [latex]4\text{NO}_2(thou)\;+\;six\text{H}_2\text{O}(g)\;{\rightleftharpoons}\;iv\text{NH}_3(g)\;+\;7\text{O}_2(1000)[/latex]

    (a) What is the expression for the equilibrium abiding (M_c ) of the reaction?

    (b) How must the concentration of NH₃ change to achieve equilibrium if the reaction caliber is less than the equilibrium constant?

    (c) If the reaction were at equilibrium, how would a decrease in pressure (from an increase in the volume of the reaction vessel) bear upon the pressure of NO₂?

    (d) If the modify in the pressure level of NO₂ is 28 torr as a mixture of the four gases reaches equilibrium, how much will the force per unit area of O₂ alter?

46. The bounden of oxygen by hemoglobin (Hb), giving oxyhemoglobin (HbO₂), is partially regulated by the concentration of H₃O⁺ and dissolved CO_ii in the blood. Although the equilibrium is complicated, it can exist summarized equally

    [latex]\text{HbO}_2(aq)\;+\;\text{H}_3\text{O}^{+}(aq)\;+\;\text{CO}_2(g)\;{\rightleftharpoons}\;\text{CO}_2\;-\;\text{Hb}\;-\;\text{H}^{+}\;+\;\text{O}_2(g)\;+\;\text{H}_2\text{O}(l)[/latex]

    (a) Write the equilibrium constant expression for this reaction.

    (b) Explain why the product of lactic acid and CO_ii in a muscle during exertion stimulates release of O_two from the oxyhemoglobin in the blood passing through the muscle.

47. The hydrolysis of the sugar sucrose to the sugars glucose and fructose follows a first-order rate equation for the disappearance of sucrose.

    [latex]\text{C}_{12}\text{H}_{22}\text{O}_{xi}(aq)\;+\;\text{H}_2\text{O}(50)\;{\longrightarrow}\;\text{C}_6\text{H}_{12}\text{O}_6(aq)\;+\;\text{C}_6\text{H}_{12}\text{O}_6(aq)[/latex]

    Rate = chiliad[C₁₂H₂₂O_eleven]

    In neutral solution, k = 2.i × 10⁻¹¹/s at 27 °C. (Equally indicated by the rate constant, this is a very wearisome reaction. In the human torso, the rate of this reaction is sped upwards by a type of goad chosen an enzyme.) (Note: That is not a error in the equation—the products of the reaction, glucose and fructose, take the same molecular formulas, C₆H₁₂O_vi, but differ in the arrangement of the atoms in their molecules). The equilibrium constant for the reaction is 1.36 × 10⁵ at 27 °C. What are the concentrations of glucose, fructose, and sucrose after a 0.150 M aqueous solution of sucrose has reached equilibrium? Think that the activity of a solvent (the effective concentration) is 1.

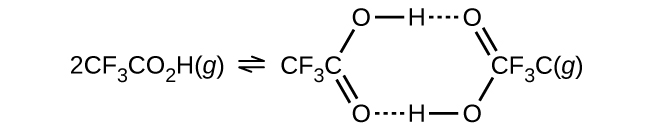
48. The density of trifluoroacetic acrid vapor was determined at 118.1 °C and 468.v torr, and found to be ii.784 g/L. Calculate 1000_c for the association of the acid.
    
49. Liquid Northward_twoO₃ is night blue at low temperatures, just the color fades and becomes green at higher temperatures as the compound decomposes to NO and NO_ii. At 25 °C, a value of K_P = 1.91 has been established for this decomposition. If 0.236 moles of N_twoO_three are placed in a 1.52-L vessel at 25 °C, calculate the equilibrium partial pressures of Due north₂O₃(k), NO₂(yard), and NO(g).
50. A i.00-L vessel at 400 °C contains the following equilibrium concentrations: N₂, one.00 M; H₂, 0.50 M; and NH_iii, 0.25 Thou. How many moles of hydrogen must be removed from the vessel to increase the concentration of nitrogen to 1.i Grand?
51. A 0.010 Thou solution of the weak acid HA has an osmotic pressure (see chapter on solutions and colloids) of 0.293 atm at 25 °C. A 0.010 K solution of the weak acid HB has an osmotic pressure of 0.345 atm under the aforementioned weather.

    (a) Which acrid has the larger equilibrium abiding for ionization

    HA [latex][\text{HA}(aq)\;{\rightleftharpoons}\;\text{A}^{-}(aq)\;+\;\text{H}^{+}(aq)][/latex] or HB [latex][\text{HB}(aq)\;{\rightleftharpoons}\;\text{H}^{+}(aq)\;+\;\text{B}^{-}(aq)][/latex]?

    (b) What are the equilibrium constants for the ionization of these acids?

    (Hint: Recollect that each solution contains iii dissolved species: the weak acrid (HA or HB), the conjugate base (A⁻ or B⁻), and the hydrogen ion (H⁺). Call up that osmotic pressure (similar all colligative backdrop) is related to the total number of solute particles. Specifically for osmotic force per unit area, those concentrations are described past molarities.)

Solutions

Answers to Chemistry End of Chapter Exercises

i. [latex]K_c = \frac{[\text{C}]^2}{[\text{A}][\text{B}]^two}[/latex]. [A] = 0.ane M, [B] = 0.1 Chiliad, [C] = 1 1000; and [A] = 0.01, [B] = 0.250, [C] = 0.791.

3. One thousand_c = 6.00 × 10⁻ⁱⁱ

v. 1000_c = 0.50

vii. The equilibrium equation is
K_P = 1.nine × 10³

9. K_P = 3.06

11. (a) −210, twox, −0.250 M, 0.250 Thou; (b) ivx, −2x, −610, 0.32 M, −0.xvi Grand, −0.48 Yard; (c) −iix, iiix, −l torr, 75 torr; (d) x, − x, −310, 5 atm, −5 atm, −15 atm; (east) x, 1.03 × 10⁻⁴ Yard; (f) ten, 0.one atm.

13. Activities of pure crystalline solids equal one and are constant; however, the mass of Ni does change.

15. [NH₃] = ix.1 × 10⁻² One thousand

17. P _BrCl = 4.ix × ten⁻² atm

xix. [CO] = ii.0 × 10⁻⁴ Chiliad

21. [latex]P_{\text{H}_2\text{O}} = 3.64\;\times\;ten^{-3}\;\text{atm}[/latex]

23. Summate Q based on the calculated concentrations and run into if information technology is equal to K_c . Because Q does equal 4.32, the system must be at equilibrium.

25. (a) [NO_ii] = i.17 × 10⁻³ M
[N_twoO₄] = 0.128 M
(b) Percent error [latex]= \frac{five.87\;\times\;x^{-4}}{0.129}\;\times\;100\% = 0.455\%[/latex]. The alter in concentration of N₂O_four is far less than the 5% maximum immune.

27. (a) [H₂S] = 0.810 atm
[H_two] = 0.014 atm
[S_ii] = 0.0072 atm
(b) The 2x is dropped from the equilibrium adding because 0.014 is negligible when subtracted from 0.824. The percent mistake associated with ignoring iiten is [latex]\frac{0.014}{0.824}\;\times\;100\% = i.seven\%[/latex], which is less than allowed by the "5% exam." The error is, indeed, negligible.

29. [PCl₃] = 1.fourscore One thousand; [PC₃] = 0.195 Chiliad; [PCl₃] = 0.195 1000.

31. [NO_two] = 0.19 G
[NO] = 0.0070 M
[O_ii] = 0.0035 M

33. [latex]P_{\text{O}_3} = 4.9\;\times\;10^{-26}\;\text{atm}[/latex]

35. 507 m

37. 330 k

39. (a) Both gases must increase in pressure level.
(b)[latex]P_{\text{N}_2\text{O}_4} = 8.0\;\text{atm\;and}\;P_{\text{NO}_2} = 1.0\;\text{atm}[/latex]

41. (a) 0.33 mol.
(b) [CO]^two = 0.50 1000 Added H₂ forms some water to compensate for the removal of water vapor and as a upshot of a shift to the left after H₂ is added.

43. [latex]P_{\text{H}_2} = 8.64\;\times\;x^{-eleven}\;\text{atm}[/latex]
[latex]P_{\text{O}_2} = 0.250\;\text{atm}[/latex]
[latex]P_{\text{H}_2\text{O}} = 0.500\;\text{atm}[/latex]

45. (a) [latex]K_c = \frac{[\text{NH}_3]^4[\text{O}_2]^vii}{[\text{NO}_2]^four[\text{H}_2\text{O}]^vi}[/latex]. (b) [NH₃] must increase for Q_c to accomplish M_c . (c) That subtract in pressure would decrease [NO₂]. (d) [latex]P_{\text{O}_2} = 49\;\text{torr}[/latex]

47. [fructose] = 0.15 M

49. [latex]P_{\text{N}_2\text{O}_3} = 1.90\;\text{atm\;and}\;P_{\text{NO}} = P_{\text{NO}_2} = ane.xc\;\text{atm}[/latex]

51. (a) HB ionizes to a greater degree and has the larger K_c .
(b) K_c (HA) = 5 × x⁻⁴
K_c (HB) = 3 × x⁻ⁱⁱⁱ

tomlinpinuplanst.blogspot.com

Source: https://opentextbc.ca/chemistry/chapter/13-4-equilibrium-calculations/

Share this post